Image size on the CCD

In order to calculate proper sampling, we will need to calculate what's the size of our target on the CCD given the focal length. Let's use the sun as an example, the sun is about 33 arc minute in size, so we have the following simple diagram:



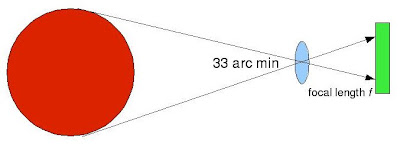
Of course, it's not on scale, but anyway. Now, suppose we have a lens of focal length f and with simple trigonometry, we can find the image of the sun on the CCD.



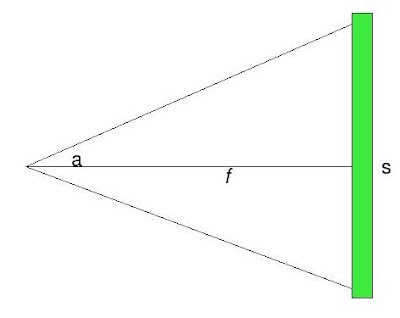
Angle a equals to 33 arc minutes as its vertically opposite angle shown in the first diagram. So, the image size s on the CCD is can be found by solving:-

tangent (a/2) = (s/2) / f

So, let's plug in the figures, for example, the image size of the solar disc on the CCD, with my Borg 45ED II which has 325mm focal length:-

tangent (33 arc minute / 2) = (s/2) / 325

s = 3.1198mm

It's pretty small, right? What's the size in terms of pixel? For example, my DMK31AF03 has 4.65um pixels, and thus, the solar disc will cover:

3.1198mm / 4.65um = 670.9 pixels

i.e. the full size solar image will be 670.9 in size at prime focus. With a 2x barlows, it will become 1341.8 pixels.